by ·0 Comments

Edit 14/03/2017: I’ve added the block contents of the two modules in another post, so you can finally compare which concepts you do or don’t have.

The question of which maths module to take is one that comes up a fair bit for students of the Open University, especially in STEM degrees.  It comes up so often, in fact, that the OU has a site devoted to the question.  For most people, this will mean choosing between MU123 (Discovering Mathematics) and the more difficult MST124 (Essential Mathematics I).

For some degrees, such as Maths, Physics, or Engineering degrees, the question is merely one of where you should start, as you’ll likely need to take and pass MST124 anyway at some point along your path. But for other degrees, you’re simply required to take a maths class, and which of the two you choose is completely up to you, with no effect on your degree or its classification.

As they’re Stage 1 modules, your pass level won’t affect classification. However, MU123 has a basic pass/fail structure, while MST124 allows the awarding of a distinction. I wouldn’t think this at all important, but someone pointed out that if they apply for a job while still on the degree course, it might be nice to say they passed all Stage 1 modules with a distinction.

I’ve mentioned earlier that I’m A) an immigrant, and B) a drop-out, so that makes deciding which maths to go into more difficult.  I was in advanced maths when I dropped out of high school early.  This would have meant I finished with the same amount of maths as non-advanced maths, but still beyond the compulsory amount required for all students. But I also skipped a year of maths before that, and had to self-teach some. So I’m left trying to match that up with “GCSE Maths” and “A-Level Maths” without anybody realising that the curriculum changes from time to time.  It would be so much easier if they simply said which mathematical concepts you needed to be familiar and comfortable with.

When I took the practice quizzes at the above mentioned site, I breezed through the MU123 quiz. When I took the MST124 quiz, I did alright through the first half of the questions, but it was taking me forever to remember formulas and rules I haven’t used in twenty years.  And the questions just felt tedious.  And I figured I just didn’t need that in my life.  So I didn’t even complete it.

Since it doesn’t make a difference to my degree, and it will be easier for me to get through and not burn me out, I was all set to simply take MU123 next year and never look back.  Working in the industry for as long as I have, I’m fairly certain it won’t ever come up in my job.

That was, however, before I encountered the Hitbox.

On a great series of MOOCs that I’m doing, I’m currently coding some graphics programmes. In all of the practice programmes we’re assigned so far, it asks us to only concern ourselves with the center point of the image, and pretend that if the image wanders halfway off the side of the screen, that’s still within the boundary of the screen.

I wanted to do it a little more advanced.  For instance, if the image is approaching the edge of a screen at a right angle, it can get as close as 1/2 the image size distance between the center-point and the screen boundary.  Easy enough to code that

But what if it’s approaching the boundary at an angle?  Now the corner of my image is further in the X or Y coordinate than half the image height or width.  How do I figure that out?

Well, it’s simple trigonometry.  As I mentioned the other week, I was self-taught in trig until last month, so I got a close look at a practical issue to see how well I understood it.  Here’s how I sketched out my problem:

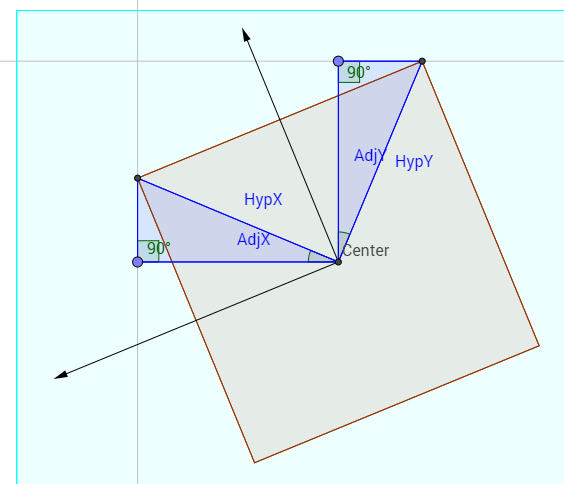
hitbox-problem

 

It’s clear to see that the closest I can get to the top edge on the Y axis is going to be the distance between the center of my image and the corner of the image (also calculated using simple trigonometry) multiplied by the cosine of the indicated angle.  Cosine(y) = Adjacent Y/Hypotenuse Y, so Hypotenuse Y * Cosine(y) = Adjacent Y.  Similarly, I need that same hypotenuse (all corners will be the same distance away from the center in a square or rectangular image) multiplied by Cosine(x) to determine how close I can get along the X axis.

So that’s all pretty basic-level maths.  But it’s a very basic hitbox, too.  What if I don’t want to pretend my images are rectangles?  What if I’m having a scalene triangle interact with an irregular pentagon?  (Adding a third dimension isn’t really all that different, you just have to increase the number of checks that are made and the calculations that represent edges.)

It’s still not that difficult to calculate hitboxes, as it becomes a series of intercepting slopes being greater than or less than line segment points.  But the hitbox is just one tiny thing to calculate. And already my shortcomings in maths could have hampered a solution if I hadn’t prepared myself.

So I think I’m now edging toward MST124. To be clear, I don’t plan to go into programming, and though I’d love a proper Computer Science degree, this is as close as the Open University gets.  But I would like to have as many bases covered as possible, and not regret that I should have had more maths under my belt when I come across something I hadn’t considered in the future.  Besides, I did go back and finish the MST124 Are You Ready quiz, and it agrees that it’s a decent fit.

by ·0 Comments

I’ve done a lot of MOOCs in the last few weeks.  The vast majority of them rate “Meh” on the scale from “Ack!” to “HOLYCANIBITZ!”  At the two extremes, I feel like I should say a bit more about them, but here are a bunch of the Meh courses that are so forgettable that I don’t have much to comment on.

Course Title: English: Skills for Learning
Provider: The Open University via OpenLearn
Price: Free
Level: Introductory
Effort: Self-paced, 24 hours, estimated 8 weeks
Prerequisites: None
Completion awards: Statement of Participation, Open Badge

About the course:
This was a fair mix of instruction and practical exercises.  The information in this course is a very small subset of the information in The Good Study Guide, which I’ve previously mentioned.  The actual time on the course for me (taking the practical exercises very seriously) was about 6 hours.  If I didn’t have the Good Study Guide, it may have been a nice reference before beginning higher education.

 

Course title: Information Security
Provider: The Open University via OpenLearn
Price: Free
Level: Advanced
Effort: Self-paced, 10 hours
Prerequisites: None
Completion awards: Statement of Participation

About the course:
This course is listed as an advanced course, but I can’t for the life of me figure out how. Really, this could have been a much shorter MOOC than its realistic 90 minutes (most of which was spent choosing and listening to a podcast): CIA stands for Confidentiality, Integrity, and Accessibility, and listen to information security podcasts.  Job done.

 

Course Title: Good Brain, Bad Brain: Basics
Provider: University of Birmingham via FutureLearn
Price: Free
Level: Introductory
Effort: 9 hours over 3 weeks, commencing on set date
Prerequisites: None
Completion awards: Certificate of Achievement + Transcript (£59 + shipping) for completing 90% of course content, attempting all tests, with score of 70%, or Statement of Participation (£39 + shipping) for completing 50% of course content and attempting all tests

About the course:
Well, as the title of the course implies, this is very basic.  But it’s also very good.  It’s a concise and informative description of many of the functions and attributes of the brain.  And while very interesting, I don’t think my heart would be in it to complete the three-part series this course is a part of.  Also, I don’t think I could take it listening to those pronunciations and reading those spelling choices again.  It felt like every scientific word was deliberately pronounced wrong and spelled the least usual way.

 

Course Title: Introduction to Cyber Security
Provider: The Open University via FutureLearn
Price: Free
Level: Introductory
Effort: 24 hours over 8 weeks, commencing on set date
Prerequisites: None
Completion awards: Statement of Participation (£34 + shipping) for completing 50% of course content and attempting all tests

About the course:
This seems like it would be a good course for someone to start considering cyber-security. As these concepts are a part of my job, none of the information in it was new, and I completed the 8 week course in about three days, so maybe 10 hours or so. I like that this course doesn’t shy away from technical descriptions of digital encryption techniques, though it doesn’t describe any actual computational mechanism for any of the concepts. As it was most definitely not directed at me, I can only say that it seems good, and has all the points and more of the things I’d want to teach someone for a primer on the subject.

It needs to re-think its active learning portions, though.  Though this is an introductory course and is limited to digital information rather than general information, it’s far more useful to all learners than the “advanced” course on OpenLearn above.

by ·0 Comments

Course Title: Learn to Code for Data Analysis
Provider: The Open University via FutureLearn
Price: Free
Level: Introductory
Effort: 12 to 20 hours over 4 weeks, commencing on set date
Prerequisites: None
Completion awards: Statement of Participation (£19 + shipping) for completing 50% of course content

About the course:

I wanted to get my feet wet with some structured programming instruction, since I’m entirely self-taught up to now. (Well, not entirely. There was that half semester in Mr. Barton’s Pascal class where I tried to hack into the school network instead of listening to him drone on about methods of averaging.)  I also wanted to start learning Python.  This course seemed like a good fit.

Data Science or coding for Data Analysis is all the rage in MOOCs right now.  I found courses all over the place teaching essentially the same skills with various programming languages. Or one from Microsoft still insisting that Excel was up to the task.

This course only touches on the barest beginning concepts in coding.  It uses pre-defined contexts for Object-Oriented Programming, but doesn’t really describe how they work aside from feeding you the formula, “If you type what I type, you’ll get the answer I get.”  The syntax it teaches is almost exclusive to the problem domain of data analysis, and it doesn’t teach much outside that syntax.

One nice thing about the course is that it’s tough. It’s not one of those courses that says it takes 4 weeks, but is actually over in about four hours.  The first two weeks took me two or three hours a piece.  The third week took me nearly five hours.  The last week was well in excess of five hours, and could possibly have been ten.  It was an absolute slog, but it was very informative.  Again, though, mostly in the realm of data science.

The course information page says that the effort required is 3 hours per week, but once you join the course it says 5 hours per week.  So keep your eyes open if you’re intending to take it.

It’s not horribly helpful on its own.  It does teach about Python, and gave me enough familiarity with it to decide that I’m not a fan of many of its shortcomings. If you do need to learn coding for data analysis, this course won’t be enough to be helpful.  If you need to learn coding for reasons other than data analysis, this course won’t be enough to be helpful.  So it’s just a taster of Python in this specific context.

One odd thing of note was that there was a great number of facilitators on the course from the Open University to assist students, but they weren’t the most polite. They were a bit aggressive with any student suggesting something would be easier a different way, or that there was a shortcoming in either method or resource.  But they were helpful to those on the course, and there were a lot of them, more support than I’ve ever seen on a MOOC.

by ·0 Comments

A few weeks ago when I reviewed OpenLearn’s Succeed With Maths MOOCs, I said that there was a much better option out there.

Course Title: Various, depending on what you want to learn
Provider: Khan Academy
Price: Free
Level: Introductory, Intermediate, Advanced
Effort: Self-paced
Prerequisites: None
Completion awards: In-site badges

About the course:

Khan Academy says they’re not a MOOC.  They’re Massive in intake, Open in philosophy, Online by nature … So that just leaves Courses.  They say they’re not a MOOC since they don’t have structured, led courses with beginnings and ends.  But they do have courses.  In fact, they have several different flavours of courses.  They have subjects, missions, skills, and … well, some kind of skill grouping that isn’t explicitly defined.  It’s in the vein of a cMOOC, rather than an xMOOC.

You just drop in, and drop out, and study what you need to study.  I’m not sure if the instruction is really as fantastic as I think it is, or if it’s just presented in a way that my unique brain makeup interprets and absorbs well, but it’s fantastic for me.

In junior high school, I had a teacher who held me back from the next year’s advanced maths class, because while I’d performed well in exams, I didn’t do any of the coursework. Then in high school another teacher noticed that the rest of the class asked me to re-teach what he’d finished lecturing about every day.  His response was to force be back up into advanced maths the following year.  The result was that I skipped trigonometry entirely.

I’ve struggled through with trigonometric functions, having taught myself a few of the rules, and then using the basic blocks I knew to struggle through more advanced issues, by combining those blocks with each other or with basic algebra.  But I’ve always felt like there was a huge gap in my learning because of this, and I’ve been a little ashamed of my skills in mathematics because of that gap.  I had tried multiple books over the years to fill that gap, but didn’t really feel like it got me anywhere.

Khan Academy filled that gap within 12 days.  In fact, what it did is show me in elegant, logical steps that were effortless to take in, that I’d gotten it right all along.  But now I don’t have to re-work and re-invent trigonometry each time I tackle it; they’ve made the knowledge much more accessible to me.

My five-year-old son was very excited to watch my progress, and see the badges I was getting, and how my avatar was growing.  He asked me to set him up an account, too.  I did, thinking he’d just log on, have a look, and log off.  Joke’s on me.

He goes on for about an hour a day, just to play.  By play, I mean take quizzes, work through practice questions, watch videos on new maths concepts … An hour a day.  And what has he learned?

He started out doing addition within the 1-20 range, and subtracting numbers in the 1-10 range.  He has now mastered two-digit addition and subtraction, is working on three-digit, can read an analog clock with or without numbers, can read information from bar graphs and solve story problems using two bar graphs, is better at understanding the operand required from story problems than I am, and is starting on geometric principles meant for 7 to 9 year olds.  In eight days.

He advanced so much, that his school literally started sending home worksheets for the next year’s maths.  Which is something that they said at the beginning of the year that they would not do under any circumstances.  (We had called for a meeting with his teacher and the head of English Learning for his school to discuss advanced reading for him, and it was stated then that their maths curriculum was designed in such a way that they would never allow students to use maths resources from advanced years. Which upset us, but didn’t think would become an actual issue.)

In addition to maths, you can study programming, history, music, economics … A lot of things. It is an amazing self-education tool and well worth any time invested in it.

by ·0 Comments

This review will be a four-in-one.  It’s only fair to review them all together, since I took them all together in less than the time it normally takes for a single MOOC.

Course Title: Learning Online: Learning and Collaborating, Reflecting and Sharing, Managing Your Identity, and Searching and Researching
Provider: University of Leeds via FutureLearn
Price: Free
Level: Introductory
Effort: 3 hours over 2 weeks each, commencing on set date
Prerequisites: None
Completion awards: Certificate of Achievement (£39 + shipping) for completing 90% of course content and 70% on test scores, or Statement of Participation (£19 + shipping) for completing 50% of course content

About the course:

At the beginning of each of these courses, they beg people to keep in mind that the content is directed at 16 to 19 year old students still in compulsory education, on their way into University.  Since the entirety of the second course, Reflecting and Sharing, is devoted to telling students how to use social media, I somehow doubt the veracity of that claim.  The last thing a teenager needs to learn is how to use social media.

There is nearly no information in any of the four modules. The majority of chapters declare that they will teach something, then tell you to visit a website and look at help files, and then claim that they just taught you something.  I didn’t find anything in the combined courses that would be helpful to a teenager or anybody else learning online, but it did offer sage advice to use netiquette generally on the Internet.  But that’s just good advice.

Perhaps the worst aspect of the courses is that they want to crowd-source some collaborative experiences, but they just kind of throw the students in and hope one of them will be first.  The problem is that it then asks the students to evaluate what’s been done so far, when there is no so far.  It’s an empty sheet.  They should have seeded the activity with some starter data to get the ball rolling.

Most of the modules are simply bereft of content, but the third course, Managing Your Identity, has information in it about managing an online image that I simply disagree with.  In fact, all four courses make suggestions like using personal Facebook and Twitter accounts for scholastic and professional endeavours.

The only useful thing I got out of all four of the combined courses was that it motivated me to find Animaker. This is a great cloud app which allows you to create an animated video with all kinds of effects.  During the Reflecting and Sharing course, it was suggested that making and sharing videos of what you’ve learned is helpful.  Note that it didn’t suggest in what way it could possibly be helpful, it just said that it was.  It then told everybody to make a video about a few questions relating to Shakespeare.

Even though I viewed the assignment as pointless, I decided to join in.  (‘Join in’ is perhaps a misnomer, as I’m thus far the only person who’s done it.)  I feel like I should extend my best effort on these MOOCs even if I don’t see the value in it, or any particular step.  I’m glad I did.  While I still can’t imagine it will in any way come up in the course of studying a degree, I had a lot of fun making it, it turned out way better than I thought it might, and it was simple.  Once the course finishes for everybody else, I’ll scrub my personal details from it (despite the suggestions from the Managing Your Identity course), and link it below.

The total time for all four courses combined was about 7 hours, including learning how to create a video on my own.  The total amount of information I got out of the course was … Well, clearly not all Russell Group universities are alike.

2016/07/18 Edit: The course is over, so I’ve scrubbed personal details from the video presentation I did … Sort of.  Anyway, here’s the finished product:

shakespearevideo

by ·0 Comments

Even more pre-study studying!  This MOOC was the first I took on FutureLearn, an endeavour of the Open University which, so far, has been far superior to OpenLearn for me.  I have since taken a further two MOOCs there, and I’ve got at least six more in the pipe-line to finish before my course begins in October.

Course Title: Begin Robotics
Provider: University of Reading via FutureLearn
Price: Free
Level: Introductory
Effort: 12 hours over 4 weeks, commencing on set date
Prerequisites: None
Completion awards: Certificate of Achievement (£49 + shipping) for completing 90% of course content, or Statement of Participation (£39 + shipping) for completing 50% of course content

About the course:
This was my first foray into FutureLearn, and it was a great experience.

FutureLearn has all students start together on a course, but they can then progress at their own rate.  You can access all weeks’ content on the first day, and the course remains open for reference after the last day.  But by starting together, there’s a community of others along the way, with each article, discussion, video, or other step accompanied by its own forum thread. If you’re struggling with something, there’s probably someone else who is, too, and collaboration is available.  (This being about robotics, I spent most of my time sharing links to interesting solutions to challenges encountered in cybernetics.)

The first week of Begin Robotics introduced the glossary of terms used, a brief robotics timeline, and robotics simulations.  This last one captured the attention of most of the students.  A variety of robotics simulations have been programmed by the Reading team to allow you to interact with a virtual robot’s programming to test changes and observe results. The interactive nature of it was great for focusing learning, and the community of students comparing results helped even further.

The second week introduced sensors and actuators, for cybernetic observing and affecting the world around it.  Robotic ‘anatomy’ was a topic of discussion, now that sensors and actuators could give such discussion context.  More simulations followed, of course focused on utilising sensor information.

The third week was about robot-human interaction.  This included haptics, interface, psychology, and more.  The fourth week was about robotic learning, and artificial life.

The best thing about this course was its pacing, combined with its multi-track learning.  A beginner could start this course, and feel that they’ve learned a lot by the end of the course, with each week being more challenging.  A serious hobbyist could likewise start this course and feel that they’ve learned a lot by the end of the course.  The two would have learned vastly different things, and never been bored.

For beginners, though, they’ll possibly be left a bit wanting at the end of the course, however, as there’s no big, “Here’s how to start,” moment for them.  It’s just a lot of great information to keep in mind once they do start.  For hobbyists, though, there are a lot of general ideas and specific solutions to some real challenges, and very much worth the time effort.

The real time effort, however, is nowhere near the estimated 12 hours.  It was closer to five or six, and I completed it in four days or so.  I keep going back, however, to participate in the online discussions, as they’re quite good.